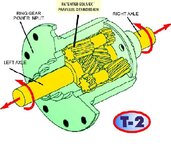
In my opinion, it is best to keep differentials and limited-slip devices as separate in your mind as possible. Yes, certain types of differentials have limited-slip devices built in, but you can still think about the two functions separately and I believe that it helps to do so. This thread will only concern the types of differentials. A separate thread will discuss the ways that slip can be limited.
The main purpose of a differential is to allow the two outputs to rotate at different speeds while still allowing each to receive at least some of the torque. Differentials are needed when the car is turning because -- assuming no tire slip -- the inside wheels turn slower than the outside wheels and the rear wheels turn slower than the front wheels. If you did not have a differential, your car would not want to turn and would suffer significant tire-wear when it was forced to turn.
There are three main types of differential. The first is the one that most people are already familiar with: the spider-type. Inside the carrier is a spider (i.e., an X-shaped piece) with at least two gears on it. The legs of the spider are firmly connected to the carrier so that the spider spins (end over end) when the carrier spins. The gears on the spider mesh with the side-gears on the output shafts. When such a diff is not differentiating, the spider- and side-gears do not rotate relative to each other. Instead, the rotation of the carrier is simply transmitted through the spider to the spider-gears to the side-gears. When differentiating, the spider-gears rotate (on the legs of the spider) while the entire carrier turns, causing one side-gear to rotate faster than the carrier and the other side-gear to rotate slower than the carrier. (In the extreme, one side-gear can be stationary and the other side-gear can turn twice as fast as the carrier.)
Side issue: Because all of the torque being transmitted from the carrier to the side-gears goes through the spider-gear to side-gear interfaces, many people upgrade cars that came with only two spider-gears to having four spider-gears, halving the force being transmitted through any one interface. In the DSM world, you will sometimes see these called "Speed Design diffs," since Speed Design makes a nice four-spider upgrade for our center differential. This is important because the center diff in an AWD DSM is one the weakest points in the drivetrain.
The second type of differential is the planetary-type. As above, the input is through the outer carrier, but in this case there are no spiders. Instead, inside the outer carrier is an inner carrier with some gears on it, and inside the inner carrier is a sun-gear. The route from the outer carrier (which has an inward-facing ring gear on it) to the sun goes like this: the outer ring meshes with a planet-gear mounted on the inner carrier. This planet meshes with another planet that is also mounted on the inner carrier. The inner planet then also meshes with the sun. This is difficult to explain without a picture (and does not appear to be well-understood by very many people), so I provide a jpg below. In this picture, the green ring is the inward-facing ring-gear on the outer carrier, the dark-blue heavy ring is the inner carrier with two light-blue planets, and the red disk is the sun-gear. Note that most planetary-type diffs have at least two pairs of planets (and usually three), but I have only included one pair because I'm a lazy sod.
The main purpose of a differential is to allow the two outputs to rotate at different speeds while still allowing each to receive at least some of the torque. Differentials are needed when the car is turning because -- assuming no tire slip -- the inside wheels turn slower than the outside wheels and the rear wheels turn slower than the front wheels. If you did not have a differential, your car would not want to turn and would suffer significant tire-wear when it was forced to turn.
There are three main types of differential. The first is the one that most people are already familiar with: the spider-type. Inside the carrier is a spider (i.e., an X-shaped piece) with at least two gears on it. The legs of the spider are firmly connected to the carrier so that the spider spins (end over end) when the carrier spins. The gears on the spider mesh with the side-gears on the output shafts. When such a diff is not differentiating, the spider- and side-gears do not rotate relative to each other. Instead, the rotation of the carrier is simply transmitted through the spider to the spider-gears to the side-gears. When differentiating, the spider-gears rotate (on the legs of the spider) while the entire carrier turns, causing one side-gear to rotate faster than the carrier and the other side-gear to rotate slower than the carrier. (In the extreme, one side-gear can be stationary and the other side-gear can turn twice as fast as the carrier.)
Side issue: Because all of the torque being transmitted from the carrier to the side-gears goes through the spider-gear to side-gear interfaces, many people upgrade cars that came with only two spider-gears to having four spider-gears, halving the force being transmitted through any one interface. In the DSM world, you will sometimes see these called "Speed Design diffs," since Speed Design makes a nice four-spider upgrade for our center differential. This is important because the center diff in an AWD DSM is one the weakest points in the drivetrain.
The second type of differential is the planetary-type. As above, the input is through the outer carrier, but in this case there are no spiders. Instead, inside the outer carrier is an inner carrier with some gears on it, and inside the inner carrier is a sun-gear. The route from the outer carrier (which has an inward-facing ring gear on it) to the sun goes like this: the outer ring meshes with a planet-gear mounted on the inner carrier. This planet meshes with another planet that is also mounted on the inner carrier. The inner planet then also meshes with the sun. This is difficult to explain without a picture (and does not appear to be well-understood by very many people), so I provide a jpg below. In this picture, the green ring is the inward-facing ring-gear on the outer carrier, the dark-blue heavy ring is the inner carrier with two light-blue planets, and the red disk is the sun-gear. Note that most planetary-type diffs have at least two pairs of planets (and usually three), but I have only included one pair because I'm a lazy sod.